MIST
Magnetosphere, Ionosphere and Solar-Terrestrial
Nuggets of MIST science, summarising recent papers from the UK MIST community in a bitesize format.
If you would like to submit a nugget, please fill in the following form: https://forms.gle/Pn3mL73kHLn4VEZ66 and we will arrange a slot for you in the schedule. Nuggets should be 100–300 words long and include a figure/animation. Please get in touch!
If you have any issues with the form, please contact This email address is being protected from spambots. You need JavaScript enabled to view it..
Particle-In-Cell Simulations of the Cassini Spacecraft's Interaction with Saturn's Ionosphere during the Grand Finale
By Zeqi Zhang (Imperial College London)
Cassini's Grand Finale at Saturn was the first time the giant planet’s atmosphere had been sampled in-situ. The ionosphere, and indeed the Saturn system as a whole, provided a uniquely different environment compared to the terrestrial planets and also Jupiter, with populations of charged dust grains influencing the plasma dynamics. When passing through Saturn’s ionosphere, Cassini observed an ionosphere dominated by ice and dust particles which continually rain inward from Saturn’s vast ring system and soak up free electrons, thus producing a dusty complex plasma. Understanding how incident plasma currents charge a spacecraft relative to its surrounding environment is important for interpreting the surrounding plasma conditions and on-board plasma measurements. In this article, we describe three dimensional Particle-In-Cell simulations of the Cassini spacecraft’s interaction with plasmas representative of Saturn's ionosphere during the Grand Finale.
The global simulations revealed complex interaction features such as a highly structured wake containing spacecraft-scale vortices and electron wings, a Langmuir wave analogue of Alfvén wings, which propagated at small angles to the magnetic field and upstream into the pristine plasma ahead of Cassini. The results explain how a large negatively charged plasma component combined with a large negative to positive ion mass ratio is able to drive the spacecraft to the observed positive potentials, a previously unexplained phenomenon observed during end-of-mission. Despite the high electron depletions, the electron properties are found as a significant controlling factor for the spacecraft potential together with the magnetic field orientation which induces a potential gradient directed across Cassini's asymmetric body. This study reveals the global spacecraft interaction experienced by Cassini during the Grand Finale in a plasma environment dominated by a class of physics quite different to those considered in the classical view of spacecraft charging.
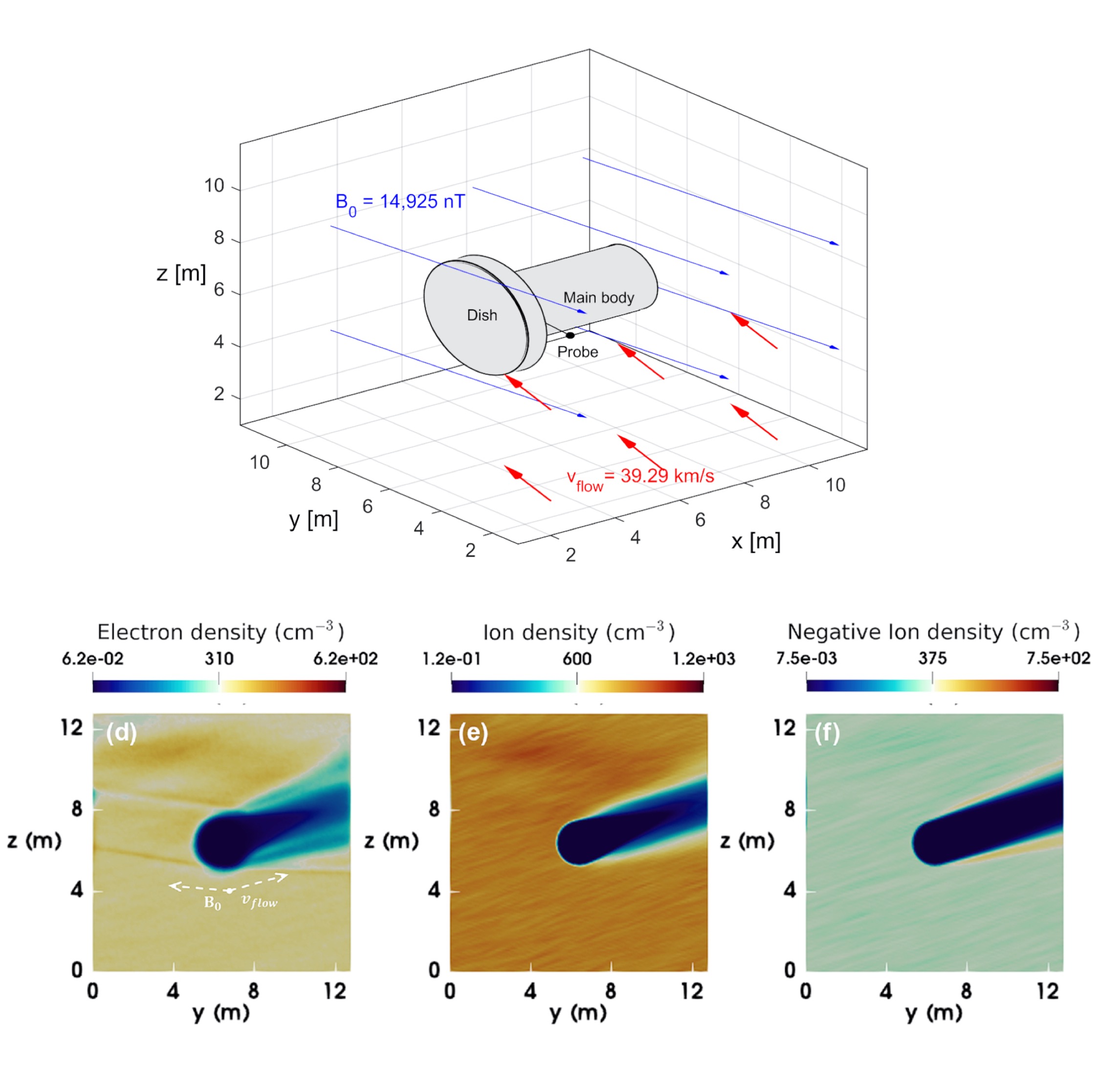
Figure 1. The upper schematic shows the simulation configuration for Cassini during Grand Finale Rev 292 ingress at 2500 km Saturn altitude. The lower panels shows the electron (left-hand panel), ion (centre panel) and negative ion (right-hand panel) densities in the Y-Z plane through Cassini’s main body. The plasma wake is longer for the larger species and electron wing structures are visible in the electron density which propagate at small angles to the ambient magnetic field.
Please see the paper for full details:
Zhang, Z., Desai, R.T., Miyake, Y., Usui, H., Shebanits, O., (2021). Particle-In-Cell Simulations of the Cassini Spacecraft’s Interaction with Saturn’s Ionosphere during the Grand Finale. Monthly Notices of the Royal Astronomical Society, Volume 504, Issue 1, pp 964 - 973, https://doi.org/10.1093/mnras/stab750.
Magnetic topology of actively evolving and passively convecting structures in the turbulent solar wind
By Bogdan Hnat (University of Warwick)
Plasma turbulence and magnetic reconnection are fundamental to the transfer of energy and momentum between field and flow and are ubiquitous in laboratory and in space plasmas. Both processes generate coherent structures, which modify the energy transfer between different scales. The precise energy balance depends on the relative prevalence of specific topological structures, their rate of evolution and their ability to carry currents.
Multi-point satellite observations of the high Mach number solar wind offer a unique opportunity to directly probe the properties of the coherent structures inherent in plasma turbulence and reconnection. We use topological invariants, nQ and nR, of the magnetic field gradient tensor to classify the topology of magnetic structures and to quantify the prevalence of actively evolving and passively advective structures and their contribution to Ohmic heating. We established that at least 25% of all samples are passively advected by the solar wind. The passive structures are dominated by plasmoids which carry a significant current density. Actively evolving structures are primarily quasi-2D flux ropes and 3D X-points. Magnetic configurations that actively evolve and carry a significant current, give a lower bound on the fraction of structures that can dissipate and heat the plasma to be ~35% of the total population. These are dominated by quasi-2D flux rope topology. Magnetic X-points constitute ~40% of all evolving structures, but only 1/5 of these carry a significant current.
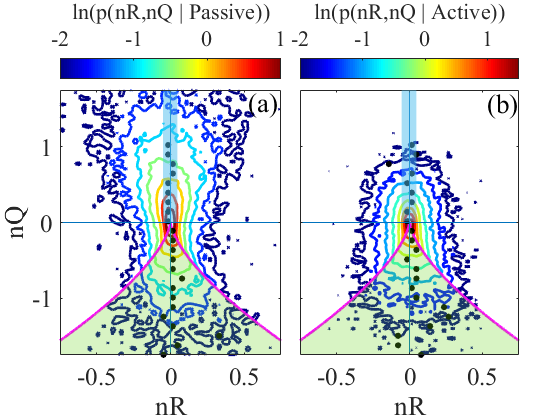
Figure 1. Conditional joint probability density for (a) force-free magnetic field, passively advecting configurations; (b) actively evolving magnetic structures. Rectangular blue shaded region with nQ>0 corresponds to quasi-2D flux ropes (O-points). Green shaded region, nQ<0 corresponds to hyperbolic 3D X-point magnetic topologies and unshaded regions represent plasmoids. Magenta line separates regions of hyperbolic and elliptic magnetic field lines.
Please see the paper for full details:
Hnat, B., Chapman, S. C., & Watkins, N. W. (2021). Magnetic Topology of Actively Evolving and Passively Convecting Structures in the Turbulent Solar Wind, Phys. Rev. Lett. 126, 125101. https://doi.org/10.1103/PhysRevLett.126.125101
Electron Bulk Heating At Saturn’s Magnetopause
By Matthew Cheng (University College London)
The magnetopause (MP) boundary is formed by the solar wind plasma flow interacting with a planetary magnetic field. Magnetic reconnection is an important process at this boundary as it energises plasma via release of magnetic energy. This process can lead to an “open” magnetosphere allowing solar wind and magnetosheath particles to directly enter the magnetosphere. At Saturn, the nature of MP reconnection remains unclear. Masters et al. (2012) hypothesised that viable reconnection under a large difference in plasma β across the MP also requires a high magnetic shear (i.e. magnetic fields either side of the boundary close to anti-parallel).
We used electron bulk heating (i.e. the scalar temperature change) at magnetopause crossings to test hypotheses about reconnection at open magnetopause locations, and the influence of magnetic shear and plasma β. The bulk temperature was determined using three different methods, related to properties of the observed energy distribution (including methods from Lewis et al. 2008). We compared the observed heating of magnetosheath electrons with the prediction based on reconnection, using the semi-empirical relationship proposed by Phan et al. (2013) which relates the degree of bulk electron heating to the inflow Alfven speed. Figure 1 shows that Δβ-magnetic shear parameter space discriminates well between events with evidence of energisation (right) and those without (left). Based on the magnetic shear measured locally by the spacecraft either side of the MP, we find 81% of events with no energisation were situated in the ‘reconnection suppressed’ regime, and up to 68% of events with energization lay in the ‘reconnection possible’ regime. These findings support the hypotheses that magnetic shear and plasma β play a role in the viability of magnetic reconnection.
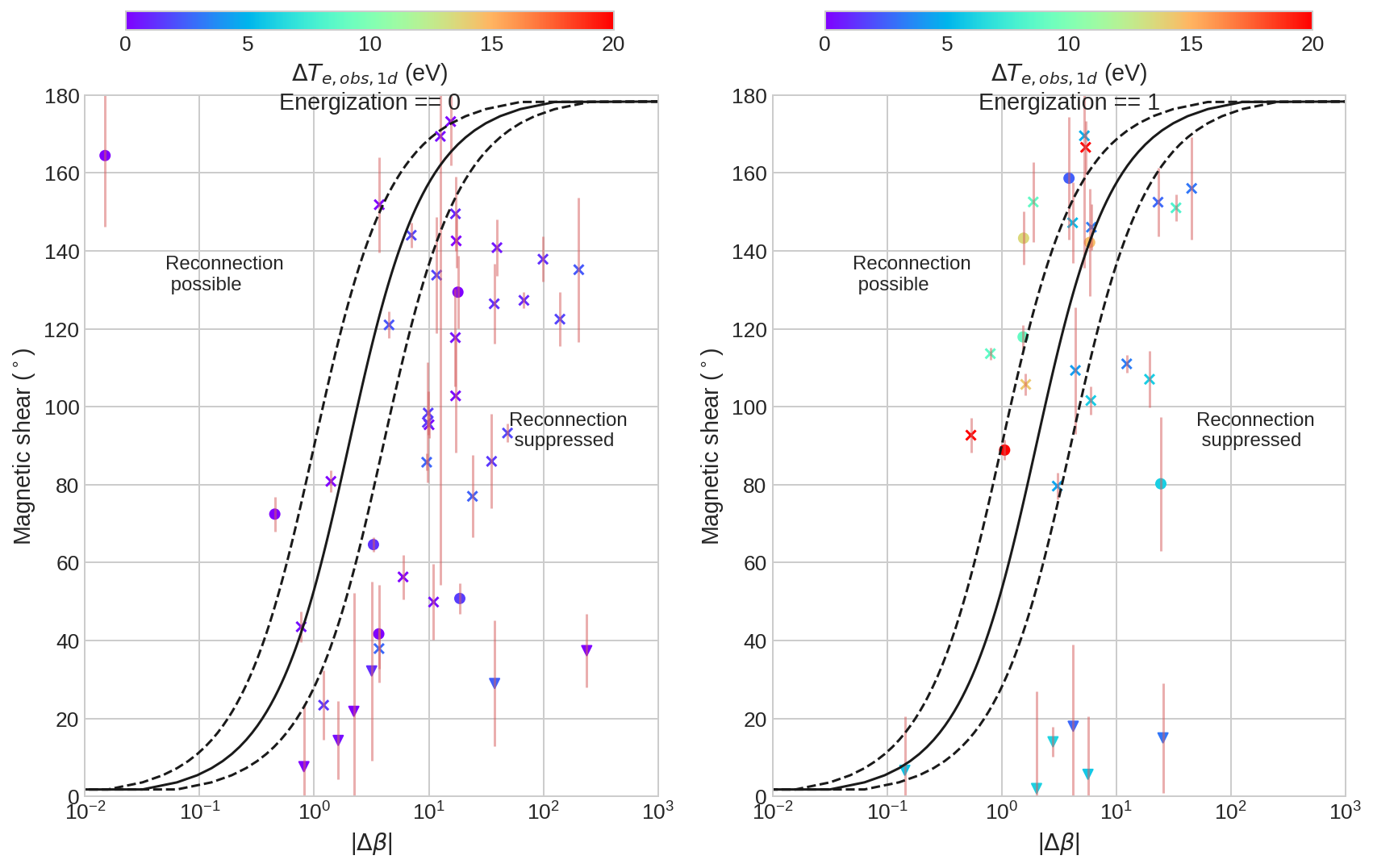
Figure 1. Assessment of diamagnetic suppression of reconnection, overlaid with electron heating ΔTe. The left and right panels show events without and with evidence of energization respectively.
Please see the paper for full details:
, , , , , & (2021). Electron Bulk Heating at Saturn’s Magnetopause. Journal of Geophysical Research: Space Physics, 126, e2020JA028800. https://doi.org/10.1029/2020JA028800
Comparing electron precipitation fluxes calculated from pitch angle diffusion coefficients to LEO satellite observations
By Jade Reidy (British Antarctic Survey)
Trapped radiation belt particles can be pitch angle scattered into the loss cone by resonant wave-particle interactions and atmospheric collisions. This high-energy electron input into our atmosphere can affect the atmospheric chemistry and is a significant loss mechanism of particles from the radiation belts, which themselves pose a threat to satellites. Reidy et al (2021) calculates the precipitating flux that would be measured inside the field of view of an electron detector on board a low earth orbiting satellite (POES) using wave particle theory and compares to in-situ data. These calculations depend on diffusion coefficients for whistler mode chorus waves, plasmaspheric hiss waves and atmospheric collisions. The diffusion coefficients used in Reidy et al (2021) were derived for use in the British Antarctic Survey Radiation Belt Model (BAS-RBM). The analysis presented in this paper is a direct test of the how well the diffusion coefficients used in the BAS‐RBM are able to quantify the precipitating flux and therefore a first step toward testing the loss due to precipitation within the BAS‐RBM itself.
Figure 1 shows a global plot of the linear correlation between the calculated precipitating flux and that measured by the POES T0 >30 keV electron channel between 26–30 March 2013. Our results show the best correlation on the dawnside for L* > 5; this agreement is consistent with chorus waves being the dominant scattering mechanism in this MLT and L-shell zone, suggesting that chorus-driven scattering is well represented in the BAS-RBM. However, we consistently underestimate the precipitating flux on the duskside, suggesting we are likely missing some diffusion here; potential causes of this underestimate are discussed in the paper. Reidy et al (2021) also demonstrates the potential of using wave particle theory to reconstruct the total precipitating flux over the entire loss cone, some of which is missed by the POES detector due to its limited field of view, finding that the total precipitating flux can exceed that measured by POES by a factor of 10.
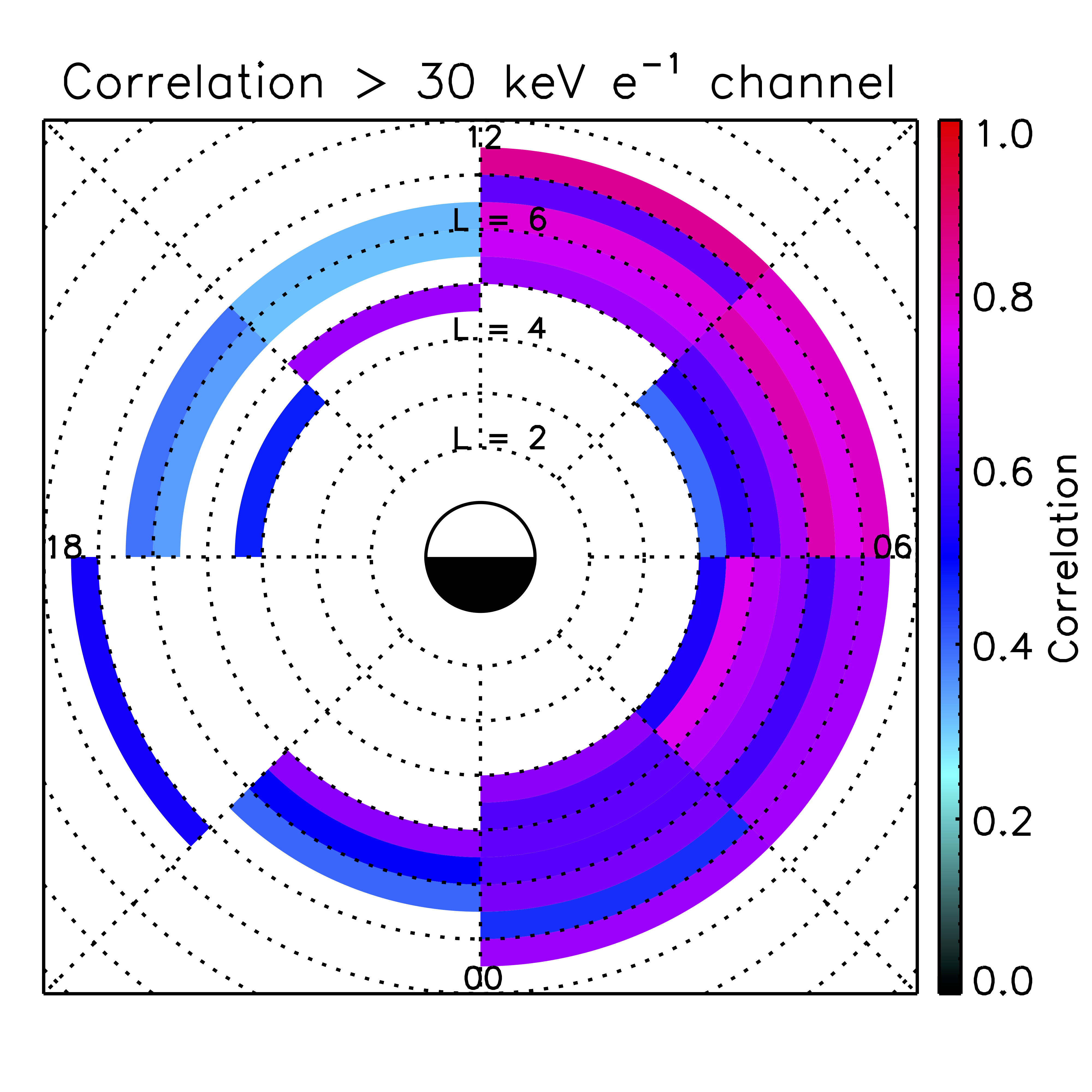
Figure 1: Linear correlation coefficient between calculated and measured precipitating flux in bins of 3 hour MLT and 0.5 L*, where noon is to the top and dawn to the right. The correlation is only shown where the confidence level is over 95%.
Please see the paper for full details:
, , , , , , et al. (2021). Comparing electron precipitation fluxes calculated from pitch angle diffusion coefficients to LEO satellite observations. Journal of Geophysical Research: Space Physics, 126, e2020JA028410. https://doi.org/10.1029/2020JA028410
Simultaneous Observation of an Auroral Dawn Storm with the Hubble Space Telescope and Juno
By Ben Swithenbank-Harris (University of Leicester)
Jupiter’s dawn storms are bright enhancements of the dawn flank of the main auroral emission, and produce the most powerful auroral events in the Solar System. These events have been observed numerous times with the Hubble Space Telescope (HST), and more recently by the Juno spacecraft, but their exact origins and related magnetospheric dynamics are not fully understood. For example, although consistent observations of this phenomena near local dawn suggested a relationship with the impinging solar wind, previous studies have shown no correlation between storm occurrence and solar wind conditions. Additionally, prior to the arrival of the Juno spacecraft at Jupiter in July 2016, auroral observations of dawn storms had not been supported by magnetospheric data from spacecraft in the dawn magnetosphere.
In this work, we present the first simultaneous magnetospheric in situ and auroral observations of the onset of a dawn storm. Magnetometer readings reveal brief reversals in the azimuthal magnetic field and decreases in the radial and total field magnitudes around the time of storm onset (Figure 1a-d). Furthermore, concurrent JADE (Figure 1e-h) and JEDI (Figure 1k-n) particle measurements reveal an increase in high energy particle populations and acceleration of magnetospheric protons towards corotational speeds, as well as long-lived hot plasma populations which persist in the outer magnetosphere beyond the expected lifetime of the enhanced auroral emissions. Ultimately, we associate this dawn storm with significant plasma heating and acceleration following reconnection at earlier local times.
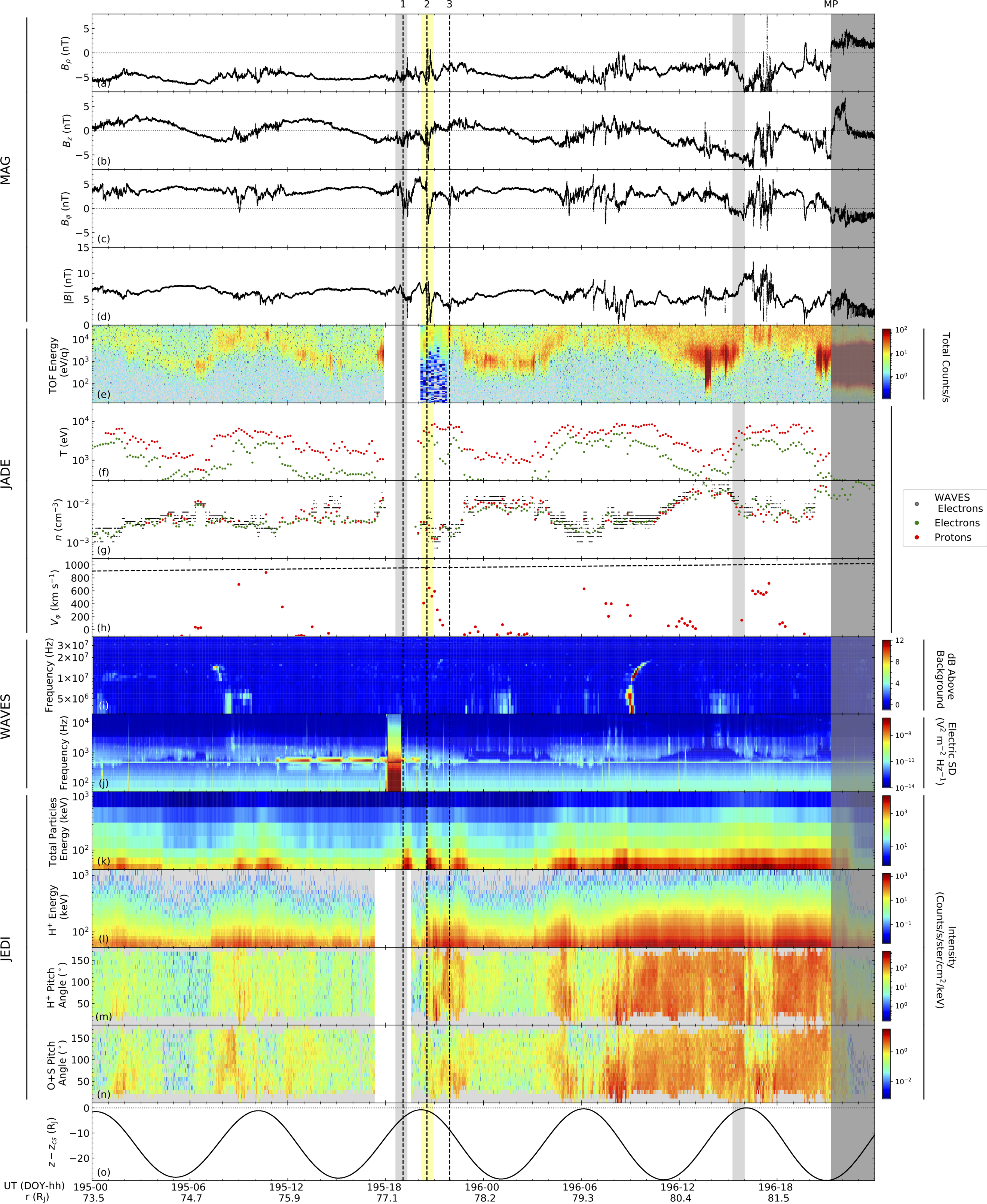
Figure 1: Overview of the Juno in situ data, showing (1a-d) the radial, north-south, azimuthal and total magnetic field strength (nT) in cylindrical polar coordinates, (1e-h) the JADE ion time-of-flight energy spectra, electron and proton temperatures (K), number densities (cm-3) and proton azimuthal velocities (km s-1), (1i-j) Waves high frequency and electric field continuum emissions, (1k-n) JEDI particle spectra showing the total particle and proton energies (k-l) and the proton and heavy ion pitch angle distributions (m-n), (1o) and the expected spacecraft distance from the centre of the current sheet (RJ), calculated using the method of Khurana (1992). The light grey shaded regions show the times of HST observations, with the dawn storm interval denoted by the yellow shaded region. The darker grey shading denotes a magnetopause crossing, and the three dotted vertical lines mark the times of several successive reversals in the azimuthal magnetic field.
Please see the paper for full details:
Swithenbank‐Harris, B.G., Nichols, J.D., Allegrini, F., Bagenal, F., Bonfond, B., Bunce, E.J., et al. (2021). Simultaneous Observation of an Auroral Dawn Storm with the Hubble Space Telescope and Juno. Journal of Geophysical Research: Space Physics, 126, e2020JA028717. https://doi.org/10.1029/2020JA028717