MIST
Magnetosphere, Ionosphere and Solar-Terrestrial
Nuggets of MIST science, summarising recent papers from the UK MIST community in a bitesize format.
If you would like to submit a nugget, please fill in the following form: https://forms.gle/Pn3mL73kHLn4VEZ66 and we will arrange a slot for you in the schedule. Nuggets should be 100–300 words long and include a figure/animation. Please get in touch!
If you have any issues with the form, please contact This email address is being protected from spambots. You need JavaScript enabled to view it..
Do Statistical models capture magnetopause dynamics during sudden magnetospheric compressions?
By Frances Staples (Mullard Space Science Laboratory, UCL)
Under steady-state solar wind conditions, the magnetopause location is described as a pressure balance between internal magnetic pressure of Earth’s magnetic field and the external dynamic pressure of the solar wind. Under extreme solar wind driving, such as high solar wind pressure or strong southward-directed interplanetary magnetic fields, this boundary is located much closer towards Earth. These compressions of the magnetopause can play a significant role in the depletion of magnetospheric plasma in the Van Allen Radiation Belts, via magnetopause shadowing. Statistical models of the magnetopause location are often used in investigations of radiation belt losses through the magnetopause. However, empirical models cannot capture the time varying nature of the magnetopause during such events, which are often associated with large step-changes in solar wind conditions.
We constructed a database of ~ 20,000 spacecraft crossings of the dayside magnetopause to assess the accuracy of the commonly used Shue et al. (1998) model. For the majority of our measurements, the Shue et al. (1998) model accurately represented the magnetopause location within an error of ± 1 RE. However, when the magnetopause was compressed below 8 RE, the model overestimated the radial distance of the magnetopause by more than 1 RE on average. This result is demonstrated in the Figure as the data does not follow the blue line, which represents where the modelled location is equal to measured location, for magnetopause measurements below 10 RE.
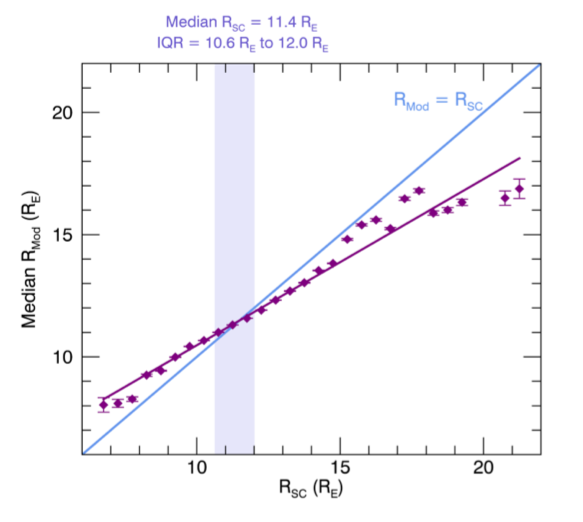
Figure: The median magnetopause location, RMod, calculated for a given measurement of the magnetopause by a spacecraft, RSC, is plotted by purple diamonds, and a best fit is shown by the purple line. The interquartile range of RSC (where 75% of magnetopause measurements were taken) is shown by the shaded region.
Furthermore, during sudden storm commencements, where interplanetary shocks impact the magnetosphere, the modelled magnetopause was significantly displaced from the measured location. Magnetopause measurements were on average 6% closer to the radiation belts, with a maximum of 42%. We conclude that statistical magnetopause parameterizations may not be appropriate during dynamic compressions of the magnetosphere and could underestimate the role of magnetopause shadowing on radiation belt dynamics. Models should be supplemented by magnetopause observations wherever possible and we have provided a dataset of THEMIS magnetopause crossing to be used by the research community.
For more information, please see the paper;
, , , , , , et al (2020). Do statistical models capture the dynamics of the magnetopause during sudden magnetospheric compressions?. Journal of Geophysical Research: Space Physics, 125, e2019JA027289. https://doi.org/10.1029/2019JA027289
The database of THEMIS and Geotail magnetopause crossings used in this study are openly available at https://doi.org/10.5281/zenodo.3700504 and https://doi.org/10.5281/zenodo.3719411 .
Using Differential Magnetometer Measurements to Monitor Geomagnetically Induced Currents in the Complex High Voltage Network of Great Britain
By Juliane Hübert (British Geological Survey, Edinburgh)
Large geomagnetic storms create time-varying magnetic fields, which induce secondary electric fields in the conductive Earth resulting in geomagnetically induced currents (GICs). The high voltage (HV) power transmission network is connected to the Earth at grounding points in substations. These offer a low-resistance path for GICs to flow into the power network, potentially causing the transformers to malfunction with extensive consequences for the national power supply. The UK government has listed severe space weather events as one of the highest priority natural hazard. Therefore, it is important to fully understand GICs to enable the mitigation of this hazard. It is possible to directly measure GICs at substations using Hall-effect probes, but due to cost and operational reasons, at present only four substations in the UK are monitored. Therefore we have developed a new instrument to measure GICs indirectly using two magnetometers, one placed under the HV line and another a few hundred metres away. By examining the differences between the magnetometers, we work out the additional current flowing in the HV line.
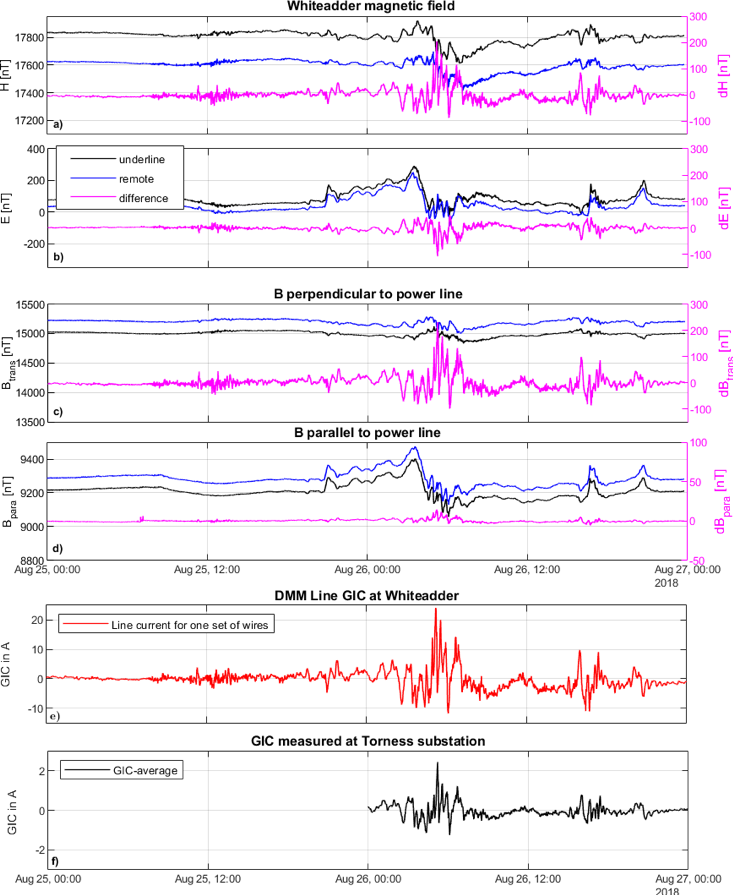
Figure 1: Recorded times series during the G3 geomagnetic storm on 25-26 August 2018. Panels a-d) Horizontal magnetic field components at DMM site Whiteadder (WHI), East Scotland. Panel e) Line GICs at WHI; Panel f) GIC data from a Hall probe at Torness substation.
In the study, we present the design and initial deployment of the first differential magnetometer method (DMM) systems in the UK and measurements from the first site installed at Whiteadder in eastern Scotland. At this site we have successfully detected geomagnetically induced currents in a 400 kV high voltage power network. The Figure compares line GIC data recorded at Whiteadder (panels a-e) to data from a Hall probe at the nearby substation at Torness (panel f) during the 26 August 2018 storm. The measured GICs from the line and the Hall probe show excellent temporal correlation, though with significant differences in amplitude, illustrating that line measurements with DMM and Hall probes at grounding points capture different but complementary views of GIC flow in a network. Using the latest model of the HV network and electric field variations estimated from a magnetotelluric survey, we show that the measured line and earthing GICs match the expected modelled values during the geomagnetic storm. This is the first study to validate such a complex network model using direct and indirect measurements of GICs.
The full article can be found here:
Hübert, J., Beggan, C. D., Richardson, G. S., Martyn, T., & Thomson, A. W. P. (2020). Differential Magnetometer Measurements of Geomagnetically Induced Currents in a Complex High Voltage Network. Space Weather, 18, https://doi.org/10.1029/2019SW002421
The Climatology of Extreme Geomagnetic Field Fluctuations
by Neil Rogers (Lancaster University)
Strong electrical currents in the Earth’s ionosphere and magnetosphere can produce geomagnetically induced currents (GIC) in ground-based infrastructure, such as electricity cables. For extreme conditions this can lead to instability and failure of the electricity supply. The magnitude of these currents is proportional to the rate of change of the horizontal geomagnetic field, dBH/dt. Climatological statistics of |dBH/dt| may be combined with models of ground conductivity and impedances in the electricity network to evaluate the risk of GICs.
Using 1.9 billion measurements from 125 magnetometers worldwide we fitted Generalised Pareto (GP) distributions to occurrences of dBH/dt above the 99.97th percentile (P99.97). By extrapolating the GP tail distributions we predicted the magnitude of dBH/dt expected every 200 years. This is shown in Figure 1a (with 95% confidence intervals) as a function of corrected geomagnetic (CGM) latitude.
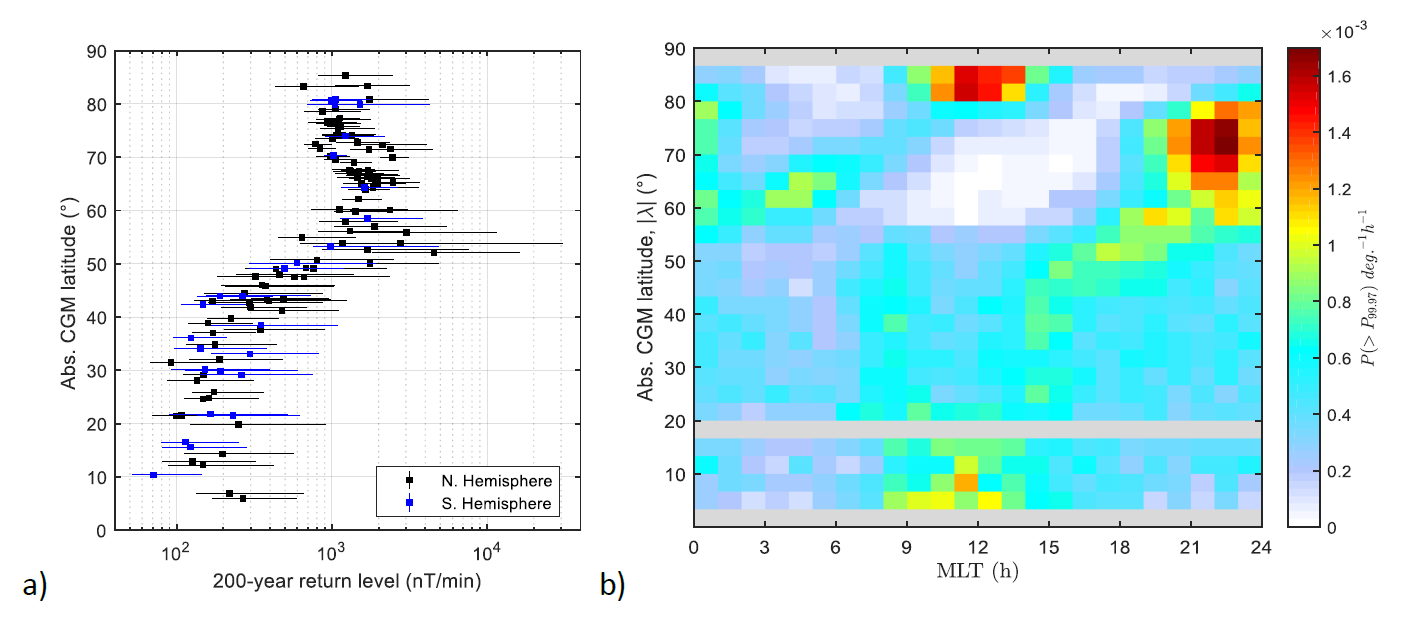
Figure 1. a) 200-year return levels for |dBH/dt|. b) Occurrence probabilities of |dBH/dt| > P99.97 vs CGM latitude and MLT.
The sharp increase near 53° CGM latitude suggests that the largest |dBH/dt| result from substorm expansions in a greatly expanded auroral region. Figure 1b presents the occurrence probability of |dBH/dt| > P99.97 vs latitude and magnetic local time (MLT). In the auroral zones this maximises in the hours before midnight due to substorm activity and in the 3-10 MLT sector due to ULF wave activity. Poleward of the dayside cusp region (~77° CGM latitude) occurrence rates increase near local noon, in summer, and under northward interplanetary magnetic field (IMF), indicating a relation to magnetospheric tail-lobe reconnection. At latitudes below 40° most occurrences were related to Sudden Commencements, the effect of shock fronts arriving in the solar wind.
This study models extreme dBH/dt as functions of latitude, MLT, month, and compass direction for return periods up to 500 years, and examines the effect of IMF orientation. The results demonstrate the response of the geomagnetic field to different drivers, and have significant potential in advancing modelling of GIC hazards.
For more information, please see the paper:
Rogers NC, Wild JA, Eastoe EF, Gjerloev JW & Thomson AWP. 2020. A global climatological model of extreme geomagnetic field fluctuations. J. Space Weather Space Clim. 10, 5. https://doi.org/10.1051/swsc/2020008
Tomographic imaging of travelling ionospheric disturbances
By Karl Bolmgren (University of Bath)
The ionosphere, the electrically charged upper atmosphere, has important effects on technologies like radio communication and satellite-based positioning. For high-accuracy positioning using Global Navigational Satellite Systems (GNSS), such as the Global Positioning System (GPS), ionospheric models are often used to estimate the ionospheric effect on satellite to ground communication. This effect is determined by the ionospheric electron content, and sudden changes or disturbances in the electron content can be challenging to include in such models.
A common type of disturbance called Travelling Ionospheric Disturbances (TIDs) are caused by gravity waves in the ionosphere, which are present all over the globe. They can be observed as wave-like fluctuations in Total Electron Content (TEC) and come in widely different spatial and temporal scales. The largest TIDs are generally caused by geomagnetic storm activity, while the more common, smaller TIDs can be caused by activity in the neutral atmosphere, like thunderstorms, perturbations from earthquakes or tsunamis, and the sudden temperature gradients associated with the solar terminator. In order to improve existing models and learn more about TIDs, we need reliable methods to study them.
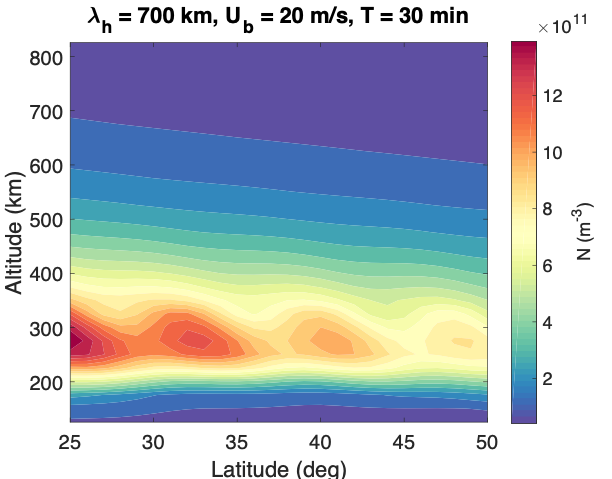
Figure: Cross-section electron density of a modelled TID used to evaluate the tomographic images. For this particular simulation, a horizontal wavelength of 700 km, an initial perturbation speed of 20 m/s, and a period of 30 min was used.
Computerised ionospheric tomography is a powerful tool to image the ionosphere. Tomography is a technique used to reconstruct the 3D structure of an object from integrated measurements and is commonly used in e.g. medical imaging. In ionospheric tomography, the 3D ionospheric electron density is reconstructed from integrated measurements of TEC. We have used simulated TIDs to test how well ionospheric tomography can be used to image different scales of TIDs, and an example of a simulated TID is shown in the figure. We showed that incorporating geostationary satellites can significantly improve the imaging of TIDs. The imaging technique has significant implications for how we observe and investigate ionospheric features, such as TIDs, and presents a method to incorporate these phenomena into existing ionospheric delay correction techniques for applications like GNSS.
For more in details, please see:
Bolmgren, K., Mitchell, C., Bruno, J., & Bust, G. (2020). Tomographic imaging of traveling ionospheric disturbances using GNSS and geostationary satellite observations. Journal of Geophysical Research: Space Physics, 125, e2019JA027551. https://doi.org/10.1029/2019JA027551
Local Time Asymmetries in Jupiter's Magnetodisc Currents
By Chris Lorch (Lancaster University)
Jupiter’s large, rapidly rotating magnetosphere is highly influenced by the presence of a global, centrifugally confined current disk comprised of co-rotating plasma from the volcanic moon Io. Azimuthal and radial currents flow through this current disk closing via magnetic field aligned currents (FACs), which are associated with Jupiter’s main auroral emission. These currents arise from dynamical processes within the magnetosphere, driven by the transport and circulation of Iogenic plasma. Characterising the structure and asymmetries in this current system is key to deciphering the dominant drivers of Jupiter’s magnetosphere-ionosphere (MI) coupled system and the behaviour of its plasma disk.
Previous work by Khurana [2001] examined the solar wind influence on Jupiter’s magnetosphere using equatorial maps of Jupiter’s current disk and the results demonstrated clear azimuthal asymmetries fixed with local time (LT). However, the analysis was limited the lack of spacecraft coverage in the dusk – dayside magnetosphere provided by the Galileo spacecraft.

Figure 1: Equatorial maps detailing the structure of Jupiter’s current disk with Jupiter located at (0,0). Dashed circles and radial lines represent 20RJ and 1 hr local time boundaries respectively. A compressed bow shock and magnetopause (Joy et al [2002]) are shown as black solid lines. a) The radial height-integrated current density, warmer (cooler) colours are indicative of current flowing radially outwards (inwards). b) The azimuthal height integrated colour density, flowing in the direction of corotation. c) The divergence of the perpendicular height-integrated current density, indicating the location of upward and downward FACs. Warmer (cooler) colours indicate the presence of upward (downward) FACs.
Lorch et al. [2020] determines the structure of the current disk at all LTs by considering magnetometer data at Jupiter from every available spacecraft, including Juno, up to 28 July 2018. We apply an automated identification tool to magnetometer data from Jupiter’s current disk in conjunction with updated models of Jupiter’s intrinsic magnetic field and current disk geometry. In total, we identify 7382 lobe traversals, calculating the associated height-integrated current density for each crossing. Additional coverage provided by the later half of the Galileo mission and the recent Juno mission allowed us to map all LTs. Asymmetries exist in both the radial (Figure 1a) and azimuthal (Figure 1b) currents into 20 RJ. Furthermore, we quantify the structure of upward and downward FACs in previously unmapped regions of Jupiter’s magnetosphere (Figure 1c). We find a positive net current density of 1.87 MA / RJ2, suggesting unmapped currents must close either down-tail or along the magnetopause. Our results demonstrate important asymmetries in Jupiter’s current systems that play a crucial role in the MI coupled system. Amalgamating these results into future MI coupling models has the potential to remove discrepancies between model predictions and observations.
For more information, please see the paper:
Lorch, C. T. S., Ray, L. C., Arridge, C. S., Khurana, K. K., Martin, C. J., & Bader, A. (2020). Local time asymmetries in Jupiter's magnetodisc currents. Journal of Geophysical Research: Space Physics, 125, e2019JA027455. https://doi.org/10.1029/2019JA027455